- Question
The arithmetic mean of all the distinct numbers that can be obtained by rearranging the digits in 1421, including itself, is
a)4444
b)3333
c)2592
d)2442
e)2222
Explanation
SOLUTION[E]-
To find the arithmetic mean of all the distinct numbers that can be obtained by rearranging the digits in 1421,we first need to determine all the possible permutations of the digits.Then,we’ll calculate the sum of these permutations and divide by the total number of permutations to find the mean.
The number 1421 has 4 distinct digits:1,2,4, and 2(repeated).
Total number of permutations=4!2!2!4!(since ‘2’ is repeated)=12
Now, let’s list all the distinct permutations:
1241
1214
1421
1412
2141
2114
2411
2411
4121
4112
4211
4211
Now,calculate the sum of these permutations:
Sum=1241+1214+1421+1412+2141+2114+2411+2411+4121+4112+4211+4211=27170
Finally,calculate the mean:
Mean=�������� ������ �� ������������Total number of permutationsSum
= 27170121227170≈ 2264.167
The closest option to this mean is:b. 2222
So,the correct answer is (b) 2222.
Question-2
The mean of six positive integers is 15. The median is 18, and the only mode of the integers is less than 18. The maximum possible value of the largest of the six integers is
a)28
b)26
c)30
d)34
e)32
Explanation
SOLUTION[E]-
Mean of the six numbers = 15
So, the sum of the numbers = 15*6 = 90
As the median is 18, the mean of middle two numbers must be 18 and thus, their sum must be 36.
Also, the mode is a number less than 18. So, the mode must be appearing as the first and the second number of the six given integers, when arranged in ascending order. To maximize the largest integers,
the mode must be equal to 1.
Therefore, out of the six integers, two are 1 and 1.
For the middle two numbers whose sum is 36, we cannot have 18 and 18 because then we will have two modes which is inappropriate as per the question.
So, the middle numbers must be 17 and 19.
The fifth integer can be 20.
Maximum possible value of the largest integer = 90-(1+1+17+19+20) = 32
Hence, option E is the correct answer.
Question-3
Twenty women can do a work in sixteen days. Sixteen men can complete the same work in fifteen days. Determine the ratio of the capacity of doing said work of a man and a woman?
a)7:4
b)5:4
c)5:3
d)3:4
e)4:3
Explanation
SOLUTION[E] (20×16) woman can complete the work in 1 day
∴ 1 woman’s 1 day’s work =1/320
(16×15) men can complete the work in 1 day
∴ 1 man’s days’s work =1/240
So, required ratio =1/24:1/320=4:3
Question-4
P can complete a work in 12 days working 8 hours a day. Q can complete the same work in 8 days working 10 hours a day. If both P and Q work together, working 8 hours a day, in how many days can they complete the same work?
a)48/7 days
b)61/11 days
c)60/11 days
d)72/11 days
e)71/11 days
Explanation
SOLUTION[C]-
P can complete the work in (128) hrs = 96 hrs
Q can complete the work in (8*10) hrs=80 hrs
Therefore, P’s 1 hour work=1/96 and Q’s 1 hour work= 1/80
(P+Q)’s 1 hour’s work =(1/96) + (1/80) = 11/480. So both P and Q will finish the work in 480/11 hrs
Therefore, Number of days of 8 hours each = (480/11) (1/8) = 60/11
Question-5
If:
A can do a piece of work in 4 hours; B and C together can do it in 3 hours, while A and C together can do it in 2 hours. Then determine as to how long will B alone take to do the same work?
a)6 hours
b)4 hours
c)8 hours
d)12 hours
e)10 hours
Explanation
SOLUTION[D]-12
Work done = 1/Time Or
Work done = Efficiency × Time
Calculation:
A can do a piece of work in 4 hours.
A’s 1 day work = 1/4
A and C together can do it in 2 hours.
(A + C)’s 1 day work = 1/2
C’ s1 day work = 1/2 – 1/4 = 1/4
B and C together can do it in 3 hours.
(B + C)’ s 1 day work = 1/3
B’s 1 day work = 1/3 – 1/4 = 1/12
Time taken by B to complete the work = 12 days
.. Time taken by B to complete the work is 12 days.
Question-6
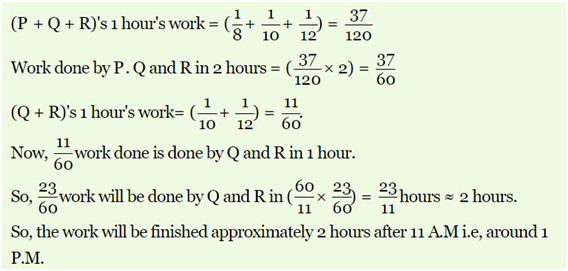
Machine P can print one lakh books in 8 hours, Machine Q can print the same number of books in 10 hours while Machine R can print them in 12 hours. All the machines are started at 9 A.M. while machine P is closed at 11 A.M. and the remaining two machines are used to complete the remaining work. Approximately at what time will the work (to print one lakh books) be finished?
a)11 PM
b)12 Noon
c)1 PM
d) 11:30 AM
e)2 PM
Explanation
SOLUTION{C]-1PM